Jumat, 02 Oktober 2009

Termodinamika


5.3 Combined First and Second Law Expressions

The first law, written in a form that is always true:

$\displaystyle dU =dQ -dW.$

For reversible processes only, work or heat may be rewritten as

$\displaystyle dW=PdV,$

$\displaystyle dQ=TdS.$

Substitution leads to other forms of the first law true for reversible processes only:

$\displaystyle dU=dQ-\underline{PdV}, \textrm{ substituted for a reversible }dW$

$\displaystyle dU=\underline{TdS}-dW, \textrm{ substituted for a reversible }dQ.$

(If the substance has other work modes, e.g., stress, strain,

$\displaystyle dU =dQ -PdV -XdY,$

where $ X$ is a pressure-like quantity, and $ Y$ is a volume-like quantity.)

Substituting for both $ dW$ and $ dQ$ in terms of state variables,

$\displaystyle dU =TdS -PdV \qquad\textrm{Always true.}$

The above is always true because it is a relation between properties and is now independent of process.

In terms of specific quantities:

$\displaystyle du =Tds -Pdv \qquad\textbf{Combined first and second law (a) or Gibbs equation (a).}$

The combined first and second law expressions are often more usefully written in terms of the enthalpy, or specific enthalpy, $ h = u + Pv$ ,

$\displaystyle dh$$\displaystyle =\underline{du}+Pdv+vdP$
$\displaystyle =\underline{Tds -Pdv} +Pdv + vdP,\textrm{ using the first law.}$
$\displaystyle dh$$\displaystyle =Tds + vdP.$

Or, since $ v = 1/\rho$ ,

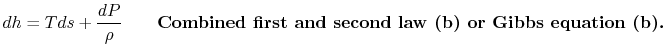
$\displaystyle dh=Tds + \frac{dP}{\rho} \qquad\textbf{Combined first and second law (b) or Gibbs equation (b).}$

In terms of enthalpy (rather than specific enthalpy) the relation is

$\displaystyle dH =TdS +VdP.$

Disusun Ulang Oleh:

Arip Nurahman

Pendidikan Fisika, FPMIPA. Universitas Pendidikan Indonesia

&

Follower Open Course Ware at MIT-Harvard University. Cambridge. USA.


Materi kuliah termodinamika ini disusun dari hasil perkuliahan di departemen fisika FPMIPA Universitas Pendidikan Indonesia dengan Dosen:

1. Bpk. Drs. Saeful Karim, M.Si.

2. Bpk. Insan Arif Hidayat, S.Pd., M.Si.

Dan dengan sumber bahan bacaan lebih lanjut dari :

Massachusetts Institute of Technology, Thermodynamics

Professor Z. S. Spakovszk, Ph.D.

Office: 31-265

Phone: 617-253-2196

Email: zolti@mit.edu

Aero-Astro Web: http://mit.edu/aeroastro/people/spakovszky

Gas Turbine Laboratory: home

Ucapan Terima Kasih:

Kepada Para Dosen di MIT dan Dosen Fisika FPMIPA Universitas Pendidikan Indonesia

Semoga Bermanfaat
Diposting oleh di
Label:

Tidak ada komentar:

Posting Komentar

Langganan: Posting Komentar (Atom)